Casino game theory
New casino sites to play real money
While decision theory is concerned with quantities and probabilities, game theory has to contend with duelling priorities and uneven, possibly unfair outcomes. It's a complex, fun field involving messy psychology, messy motivations and even messy thinking with economics and cuts to the heart of human nature. Let's return to dinner. This time you're eating solo, deciding between your go-to local bar and a gourmet sushi place.
Distrust your gut
Game theory for everyday decisions
Introduction
In the immortal words of kenny rogers: you gotta know when to hold вђ˜em, know when to fold вђ˜em, know when to walk away, know when to run.
Itвђ™s as true in gambling as it is life: from what you wear to the person you marry, your life is the result of your choices. How can you be sure youвђ™re making the right ones?
Itвђ™s dizzying to think about all the factors that go into even small decisions.
Take one of lifeвђ™s least dramatic scenarios: dinner with friends. Consider the sheer number of variables involved in such a routine dilemma:
How much is everyone willing to spend? Which restaurants are rated best? And how can you be sure the majority arenвђ™t claiming to be вђњgood with anything,вђќ while secretly loathing your final selection?
Yikes. Even in a situation this trivial, uncertainty is rampant and getting it wrong can have negative impacts on your wallet, social life or stomach. Yes, weвђ™re over analysing вђ“ but whether itвђ™s life and death or pizza versus pasta, every decision has risks and rewards, probabilities and payoffs.
The good news is that thereвђ™s plenty of work out there that breaks decision-making down into basic concepts, and mastering just a few of them will leave you better equipped in both the casino and your everyday life.
Decision theory nature vs. Numbers
There are two ways of understanding choice: going with your gut, or using your brain.
While many gamblers argue otherwise, going with your gut is a quick path to chaos вђ“ an impulsive decision-making process that tends towards either the random or the patently wrong. Using your noggin seems the more obvious choice, and doing so commits you to rational analysis instead of flying by the seat of your trousers.
Let's return to dinner. This time you're eating solo, deciding between your go-to local bar and a gourmet sushi place.
The strategies here that don't involve your gut (pun intended) draw from вђњdecision theoryвђќ, a study focused on probabilities. Decision theory recommends using basic multiplicationвђ”the value of the proposed benefit (a delicious meal) multiplied by the probability of achieving said benefit.
On a scale of 1 to 10, you'd grade the barвђ™s nachos as a 5. Sushi is among your favourite foods, so you score it a 10.
But letвђ™s say the sushi restaurant has been phenomenal on one occasion and terrible on two other visits. Your probability of a 10-point experience is just 33%. The local bar, having never let you down, is 100% likely to serve up a perfectly fine dinner.
Should you shoot for the greater reward of sushi, despite the risk?
Decision theorists call this a problem of вђњexpected utilityвђќ, where вђ˜utilityвђ™ is just used to mean simply вђ˜desirable outcomeвђ™. Your expected utility for dinner at the local bar is 5 (the value of the meal) multiplied by 100%. That comes to 5. Your expected utility for the sushi spot is 10 multiplied by 33%, or 3.3. Since 5 is greater than 3.3, decision theory would typically steer you away from the wonderful but unreliable choice and towards the decent, risk-free option - the optimal balance of risk versus reward.
Game theory
When more than one person is involved in a choice, game theory takes over. Game theory proposes that nearly any interaction between decision makers can be mapped out as a game or organized thought experiment. Wherever decisions overlap, game theory applies.
While decision theory is concerned with quantities and probabilities, game theory has to contend with duelling priorities and uneven, possibly unfair outcomes. It's a complex, fun field involving messy psychology, messy motivations and even messy thinking with economics and cuts to the heart of human nature.
A history of choice from pascal's wager to the prisoners dilemma
The roots of decision and game theory date back to 1669, when mathematician and philosopher blaise pascal presented this argument for believing in god:
When it comes to your stance on god, you have two choices: believe in god, or donвђ™t. Religion aside, whatвђ™s the rational decision?
Suppose you're dead. If god exists and youвђ™re a believer, your payoff is infinite. You go to heaven for eternity. Congratulations!
If god doesn't exist and youвђ™re a believer, your loss is finite and relatively small. In living a clean life, you enjoyed yourself less than you might have - but regrets are for the living and the afterlife, and neither now applies.
Now imagine you're a disbeliever. Your best payoff isn't much of a payoff at all: partying hard, then dying and not going to heaven, because neither it nor its supposed creator are real.
But if you disbelieve, and god does exist, your loss is infinite. You're going to hell.
Self-interest alone dictates you choose the strategy with the best possible payoff; like it or not, you should be a believer.
Pascalвђ™s wager breaks down in a world of multiple worldviews, but as pure maths (and game theory), it remains broadly relevant. We can use the same rationale to save the planet. For example: should you believe or disbelieve in the climate crisis?
Belief, and the effort that accompanies it, will either result in saving the human race or losing some time and money to an overblown problem. Disbelief might save you a finite amount of cash and effort if climate change turns out to be no big deal вђ“ or lead to humanityвђ™s untimely demise.
The game is afoot von neumann and the birth of game theory
To understand why humans are terrible at cooperating, we need to dig into the history of game theory - starting with the man who essentially invented it.
Mathematician john von neumann helped usher the era of nuclear weapons as part of the manhattan project. But before he was helping the military blow things up, he authored various works on game theory and economic behaviours.
Von neumann's status as the father of game theory is based on his cold-blooded, mathematical analysis of competitive scenarios.
Along with describing interactions between decision-makers as вђњgames,вђќ von neumann proved that in two-person вђ˜zero-sumвђ™ games, or contests where one player's gain is the other player's loss, fortune doesn't actually favour the brave. The best strategy is to minimize your losses in the event of a maximum loss situation вђ“ his вђњminimax theoremвђќ.
In gambling, that means mapping out the worst possible outcomeвђ”an opposing poker player holding the best possible cards for a given handвђ”and choosing the action that leaves you least vulnerable should that ugly outcome materialize.
More than choosing based on static probabilities - like the odds the dealer shows an ace to complete your hand вђ“ strategies for competitive games require putting yourself in your opponentвђ™s shoes.
What happens if that ace turns up and they push all of their chips in? With enough time, every possible outcome of this situation could be mapped out, including weighing the risks and rewards of folding or staying in.
By associating games with the larger world of economics, von neumann showed that most human interactions could be modelled, opening the door for smart thinkers to apply game theory to everything from the stock market to nuclear warfare. The wide-ranging implications make it no wonder that, over the years, twelve nobel prize winners have been game theorists.
Built to betray the prisoner's dilemma
In an arms race, competing nations devote precious resources to stockpiling weapons they may never use theyвђ™re responding to the actions of their enemies, choosing a merely bad outcome (huge spending on unused weaponry) to avoid a worse one (defeat at the hands of a better-equipped enemy).
This goes beyond pascalвђ™s wager, since armed conflict comes with multiple finite consequences ranging from minor battles all the way up to nuclear annihilation. To understand this interaction, we need game theoryвђ™s most famous exercise: the prisonerвђ™s dilemma.
Imagine two criminals caught for the same crime and given a choice: betray your partner (defection), or shut your mouth (cooperation).
If one defects and the other cooperates, the defector is released and the co-operator gets ten years in jail. If both criminals defect, they both receive a three-year sentence. Finally, if both criminals refuse to rat the other out, they both get one year.
Hereвђ™s the thing: if no communication is allowed between the prisoners, it becomes rational and even inevitable that both players will betray each other. Hereвђ™s how it breaks down:
If a prisoner cooperates, he faces the possibility of the game's best-case outcome (one year, if both cooperate) and worst outcome (ten years, if his partner defects). That still falls short of the game's biggest win: freedom. Coming from a place of self-interest, this is a grim path.
If a prisoner defects, he avoids the worst possible outcome (since his maximum sentence is three, rather than ten years) and has a shot at the best payoff (sweet, sweet freedom). If you're looking out for number one, this is the preferred choice.
That makes defection in the prisoner's dilemma a dominant strategy: whatever the other player chooses, the defection strategy promises a better payoff. By ratting on your partner, you're also minimizing your losses in a worst-case outcome.
Now swap nervous criminals for armament-happy nations. Suddenly the prisoner's dilemma explains the tendency for opposing powers to keep stockpiling weapons instead of levelling off early. Since both players in the game are forced to choose the dominant strategy, assuming that both sides are rational, both are going to defect. They'll act out of rational self-interest and avoid cooperation at all costs. The result is a game that's immediately locked into an escalating standoff.
The irony of the prisoner's dilemma is that the shared outcome is actually worse than it would be if both parties opted to cooperate. Opposing defectors each get three years of jail time, while co-operators would be out in a year вђ“ and the same goes for an arms race: resources are consumed and war is averted вђ“ but both nations would be better off having agreed to never waste the investment.
Game theory doesn't just show us the best choice in a given situation, it also shows us why bad choices are so hard to avoid.
Game theory in 10 easy steps


1. AS YOU MIGHT HAVE GUESSED, “GAME THEORY” IS THE MATHEMATICAL STUDY OF GAMES
But not just any type of game. Game theory concerns only games of strategy between two or more competing players. Usually there’s a clear set of rules that define the possible moves that each player can make at each juncture in the game and a resulting score for each player at the end of each play of the game.
2. DON’T CONFUSE “GAME THEORY” WITH “GAMBLING THEORY”
Although the two do overlap. Gambling theory applies to games with uncertain outcome, with one or many players, with or without any skill involved. You could say that game theory is about games of skill, and gambling theory about games of chance (although a single-player gambling game that involves some skill would not be a subject of game theory). Video poker, which involves just a single player attempting to make winning combinations, has an element of skill but it still wouldn’t be a subject for game theory. But live poker, played amongst two or more people, is a fitting subject for game theory. In fact, poker games, especially simplified versions, have been used as paradigms in game theory since john von neumann founded modern game theory back in the 1920s.
Similarly, game theory doesn’t apply to the game of blackjack, even when a skillful card counter gains an edge over the casino. But, if you consider the cat-and-mouse game that card counters have to excel at to be allowed to keep playing and beating the casino, game theory has a lot to say about that.

3. GAME THEORY SPLITS UP GAMES INTO TWO TYPES ALONG SEVERAL DIFFERENT CRITERIA
There are cooperative versus non-cooperative games, zero-sum (or more accurately, constant-sum) versus non-zero-sum games, simultaneous versus sequential games, games of perfect information versus games of imperfect information, and two-player games versus games with three or more player. There are also symmetric and asymmetric games, infinite games, combinatorial games, repeated games, discrete or continuous games, population games, stochastic games, meta-games, and so on.
It’s good to know that these terms exist, but I won’t be able to cover all of them here.
4. THE ECONOMIC PREDICTING BEHAVIOR MODEL
Game theory was originally developed as a mathematical model for studying and predicting economic behavior. But the wide variety of games that game theory contemplates makes it valuable as a model or tool for many fields, including evolutionary biology, philosophy, political science, computer science, warfare, and, of course, multi-person gambling games like poker. Remember the classic 1983 movie war-games? (if you haven’t seen it, that’s your first homework assignment.) that’s game theory applied to thermonuclear war.
“perfect information” in game theory doesn’t mean that you know the future. It only means that each player has perfect information about the past
5. I WANT TO PLAY A (ZERO SUM) GAME
A game is zero-sum if the total score at the end of each play is zero. In other words, however much one person wins will be lost by the other player(s) in the game. Gambling games like poker are usually zero-sum games (not including the house rake, but also not including the entertainment value, which is why we play in the first place). There are some games where players can combined do better or worse depending on their strategies. Games like this are used sometimes as models for how cooperation can evolve between otherwise competing players.

6. CAN YOU PREDICT THE FUTURE? THAT’S PERFECT
“perfect information” in game theory doesn’t mean that you know the future. It only means that each player has perfect information about the past (and present, if there are simultaneous moves by different players). There’s no hidden information that one player knows that another doesn’t, like a player’s hidden cards in a poker game. And there are no hidden moves, like the starting position in a game of battleship.
Games like chess, checkers, and go are games of perfect information, and there’s a separate branch of mathematics called combinatorial game theory that focuses on them. “regular” game theory focuses more on games with imperfect information. There still can be randomness and uncertainty in a game of perfect information. Backgammon is a good example, and monopoly too. (speaking of monopoly and war games, in world war II, the allies actually smuggled maps, compasses, and other tools to pows, inside monopoly boards!)
7. IT’S ALL ABOUT INELEGANCE
The main focus of game theory is on fully rational, intelligent players.
Game theory is interested in answering the question, “what’s the best way to play against opponents that think like I do?” the focus isn’t on how to play against a particular opponent with a certain strategy (although that can be a step in an algorithm for finding an optimal strategy). It’s like a genius playing against a perfect twin.
“what’s the best way to play against opponents that think like I do?” the focus isn’t on how to play against a particular opponent with a certain strategy.
8. PURE STRATEGY VS MIXED STRATEGY
In every two-player zero-sum game, like heads-up poker, there is a strategy (or a set of strategies) that cannot be beaten, even by the best opponent. That’s usually called the optimal strategy. If you follow the optimal strategy, you can even tell your opponent how you play (but don’t give away tells!) and you can’t be beaten in the long run. Optimal strategies often involve picking randomly (but with precise probabilities) between two or more different playing choices for some or all moves of the game, because an opponent can sometimes take advantage of a strategy that is too predictable. (that’s called a “mixed strategy” in game theory, as opposed to a “pure strategy” that always makes the same choice of move in identical situations.)
for example, in the game rock-paper-scissors (also called roshambo), the optimal strategy is to make each play one-third of the time. If you played rock even slightly more often than that, your opponent could have an advantage by playing paper more often than one third and/or playing scissors less often. Note that the optimal strategy in roshambo won’t give you an advantage, but it will prevent your opponent from gaining an advantage. Fortunately, that’s not true of poker. The optimal strategy, if you can figure it out and follow it perfectly, will give you an advantage against almost anyone else.
You may have heard that poker researchers “solved” a real-life poker game last year (heads-up limit texas hold’em). They didn’t literally solve the game, but they did get close enough, using thousands of days of computer time, that they felt they could say that they “essentially” solved it. You could play perfectly against it for your whole life and still have a good chance of losing.
Quote: if you follow the optimal strategy, you can even tell your opponent how you play (but don’t give away tells!) and you can’t be beaten in the long run
9. EQUILIBRIUM STRATEGIES
Add just one more player, so there are 3 players in a game (or the game isn’t zero-sum) and there isn’t guaranteed to be a “best” or “optimal” way to play. However, there can be “equilibrium strategies”, called nash equilibria, named after john nash, the famous mathematician who won the nobel prize in economics in 1994 for his groundbreaking work. Nash was the subject of the multiple-academy award-winning movie A beautiful mind, based on the book of the same name. Sadly, last year he and his wife were killed in a car accident in new jersey, being driven home from the airport after another prize-winning trip to norway.
10. GET OUT THERE AND PLAY
In a nash equilibrium, no player can do better by unilaterally changing his or her strategy. But, two or more players could change their strategy together and see an improvement. Applying that to a poker game: when there are more than 2 players in the game, there is no strategy that will always win (even when your opponents aren’t purposefully colluding against you). That means you must adapt your strategy to your opponents, and how they are playing at that time. To complicate matters, they are going to be constantly trying to adapt to you and each other too. And, sometimes, even by chance, they may be playing in a way that you can’t beat in the long run. So, find a poker game that you’re comfortable in and can win at, or seek a heads-up (two player) game.

Finally, that brings us to your final assignment: get out there and play. (tell ‘em you’re just doing your homework!)
Game theory optimal solutions and poker: A few thoughts

GTO stands for “game theory optimal.” in poker, this term gets thrown around to signal a few different concepts. It refers to thoughts about opponent modeling, and thinking about poker situations in terms of ranges and probabilities, as opposed to being strictly results oriented.
Sometimes those ideas gets reduced to young pros shouting across a poker room (or the twittersphere) about whether a given play is “GTO” — or even “the opposite of GTO,” as I recently saw in a discussion thread. But what does this really mean? And does it apply to your game?
Seeking an unexploitable strategy
A game theory optimal solution to a game has precise mathematical definitions. It is interesting to consider what this means to a poker player, as well as how this concept has become a dominant framework for looking at ideal poker strategy. Since most of my time these days is spent building computer ais that play strong poker, I’m often thinking about how computers look to GTO strategies for playing unexploitable poker.
GTO — especially in the context of modern poker — is largely about pursing a strategy that makes it impossible for you to get pushed around. Think uma thurman in kill bill. Or bruce willis in any bruce willis movie.
Outside of poker, GTO is usually introduced with the “prisoner’s dilemma.” in this hypothetical situation, the two of us are arrested for jointly committing a crime. If neither of us talks, we both get off with light sentences. However, if one of us snitches on the other, the snitch will get off with no punishment at all, while the person who doesn’t talk gets a harsh sentence. If we both snitch, we both get a harsh sentence, since each person’s testimony can be used against the other.
Even though we would be best off with the first scenario (nobody talks), each individual is better off from collaborating with the authorities, regardless of what the other does (if I don’t snitch, you should snitch to get off free, and if I do snitch, you should definitely snitch as well). In an environment where players are rewarded for taking advantage of each other, it may not be worth acting cooperatively, even if all sides would be better off by doing so.

The poker equivalent would be two players fighting it out on the bubble of a tournament. Except for the super-deep stacks who can chip up on the bubble with no risk of busting, the remaining players benefit from any confrontation that leads to an elimination. Thus the two players in the hand are only hurting themselves, by trying to bust each other. And yet, it’s not possible for them to collaborate toward a mutually beneficial solution.
Reacting to an opponent’s attempts to run you over is so natural to a thinking poker player, framing it in terms of GTO can seem almost superfluous. Of course your opponent has a strategy. You have some idea of what that strategy would be with various hands, and your job is to take that into account when executing your own strategy.
In other words, play the player. This is what GTO is all about.
The quest to “solve” hold’em (and other games)
As you adjust your strategy to an opponent’s strategy, he or she will adjust to yours, and so forth. For heads-up limit hold’em, the university of alberta team took this process to its logical conclusion, publishing their results earlier this year in science magazine. Using a network of computers, they set two strategies loose, repeatedly adjusting to each others’ play. Eventually, they reached a state where neither player could gain even a 1% advantage against the other in any specific situation.
This sounds complicated, and I’m simplifying what they did slightly. But in essence, they reached a strategy which an opponent cannot exploit — or at least cannot exploit beyond a 1% edge — with any other possible strategy. Somewhat confusingly, the university of alberta team claims both to have “solved” heads up limit hold’em, and also that they found just one GTO equilibrium for heads-up limit hold’em, and that there are likely to be other equilibria for the game, left to be discovered.

According to the paper, their “near perfect” heads-up limit hold’em bot raises 90%+ of hands on the button, but doesn’t four-bet when it gets three-bet from the BB almost at all, even with
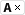
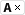
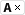
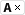
However, the alberta folks are quick to point out that calling a three-bet with
In practice, if you know that your opponent will call off with one-pair hands against aces, and not react as though he knows your very tight four-betting range, then you’re just missing a bet. In a recent episode of the thinking poker podcast, andrew brokos and nate meyvis explain this point well. Game theory uses a strong definition of optimal play, where you’re supposed to consider every play you would ever make with any hand as part of the equilibrium. However in real cases, 95% of that is optimizing for what you would do in this spot, given the range of hands that you could be playing, and what your opponents’ hands might be.
In a hand discussed on the show, a listener in a limit hold’em game held
Let’s think about this situation as a computer AI might. Say you’re playing $100/$200 limit hold’em. The pot is $400, and you raised preflop with
When everyone knows what everyone else is doing
Once you’re in three-handed (or more) games, there is no game theory optimal solution, strictly speaking. This is because there is no stable equilibrium (or too many equilibria to count, depending on whom you ask). The players can always adjust to each other, or take advantage of a player trying to execute a GTO strategy and not adjusting to them, through a process that bill chen and jerrod ankenman call “implicit collusion” in their 2006 book the mathematics of poker. Thus there is no unexploitable strategy.

Let’s dig into this for a second. When playing heads up, if you (or a bot) follows a GTO strategy, an opponent can’t beat you in the long run, no matter what he or she does. This does not mean that you are winning the most against this opponent, but you are locking in a long-term tie, while still benefitting from some of your opponent’s mistakes.
For example, the limit hold’em GTO bot will pay off on the river with bottom pair often enough so you can’t bluff it effectively. If you never bluff in this spot, the bot will still pay you off at the same rate. An exploitative player would stop paying you off after a while, and win even more. Doug polk spoke on the twoplustwo pokercast about this situation coming up during the man-vs.-machine NLH match last spring. It was such a relief to the players once they realized that while the computer played well (“4 out of 10” compared to his regular opponents, according to polk), it did not attempt to exploit their betting patterns. If when you flop the nuts you bet 1.5x the pot or crumble a cookie, the AI doesn’t know or care. It just plays GTO.
In an idealized 3+ player game where everyone adjusts to everyone, GTO should not work. But in practice, if the players don’t change their strategies too much from hand to hand (and they don’t), a lot of the heads-up GTO principles apply.
A friend of mine went to graduate school with one of the best online players in the world, and had a chance to watch him play. He was surprised that his classmate did not make any unusual plays, or really any “moves” at all. According to the pro:
- Everybody knows who I am
- Everybody knows how I play
- There’s no reason to get out of line
If you take chen and ankenman’s ideas about “implicit collusion” to heart, one could also add that if the players were ganging up on him instead of trying to beat each other, the pro would just quit the game. This is a non-issue in the nosebleed games, since everyone knows everyone else, and playing anonymously or collusively isn’t really possible.
The point is, the best player in online poker last year (on a per-hand basis) plays GTO. He must be really good at knowing when to bet 80% of the time and to call 20%, and when to call 20% and to fold 80%. And then he actually does it. There’s a lot to be said for good execution. (I tend to find that 20% call button a little bit too often.)
It’s also easy to see why polk in the same interview is pessimistic about humans’ chances, once the bots learn all the right bet frequencies. Our silicon friends will always have that edge in execution, and they don’t need room, food, or beverage.
Conclusion: GTO is the baseline
In the short term, the humans are converging on GTO more quickly. When I sat in the stands in the amazon room at the rio all-suite hotel and casino for the final table of the $1 million big one for one drop, it shocked me how loose-passive the play became after getting down to three-handed.

I took some flack on twitter for comparing the small-bet, check-down game happening between daniel negreanu, dan colman and christoph vogelsang to a nightly satellite at a local casino. Folks fired back that these guys are the best in the world, and I’m clearly an idiot. But it sure did look like none of the players were trying to pressure the others. And why should they? With payouts of $15M, $8M, $4M, there was a lot less upside in winning chips than was the downside to chipping down or busting.
On other hand, if one guy pushed, he knew the others knew how to fight back. So nobody pushed. For about two hours, three of the best short-handed NLHE players in the world checked or small-bet every hand, until vogelsang, the short stack, busted.
Do you need to play GTO in order to win? Or rather, how close do you need to get to GTO in order to hold your own against a strong set of opponents? Let’s let professor tuomos sandholm, head of carnegie mellon’s claudico no-limit hold’em team, answer that question.
In a recent article in cigar aficianado interviewing academics and enthusiasts at the annual computer poker championship, sandholm was asked about his colleagues at the university of alberta solving limit hold’em.
“they say it is essentially solved. I think that counts,” responded sandholm. “my question though is: was it essentially solved three years ago?”
Near-optimal GTO play is just the first step. Once your baseline strategy can’t be easily exploited, you can spend the rest of your time studying opponents’ tendencies and adjusting to their weaknesses. There will be plenty of opponents who don’t think about ranges, who don’t adjust to some of the game information, or who are just playing their own way. Adjusting to them is what GTO, and poker, is really all about.
For a good, accessible exploration of how to use GTO in your game, check out ed miller’s book poker’s 1%: the one big secret that keeps elite players on top . Meanwhile for examples of how players use hand ranges to adjust to their opponents’ strategies, see any of alec torelli’s “hand of the day” analyses here on pokernews, or the recent interview with vegas $2/$5 NL pro sangni zhao.
Nikolai yakovenko is a professional poker player and software developer residing in brooklyn, new york who helped create the ABC open-face chinese poker iphone app.
Want to stay atop all the latest in the poker world? If so, make sure to get pokernews updates on your social media outlets. Follow us on twitter and find us on both facebook and google+!

Poker pro and software developer nikolai yakovenko on the applications of "game theory optimal" solutions to poker.
You've heard talk of "GTO" in poker, but how do game theory optimal solutions apply to decision-making in poker?
Chaos theory: can it become a strategy at blackjack & roulette?


The law of independent trials is fundamental principle that allows the casino gaming industry to accumulate mass amounts of wealth from unsuspecting customers. Every game in the casino has the perception of being completely random in nature. This means there is no predictability of the outcome of an upcoming event. What the casinos rely on is the overall advantage that their games have that favor the casino. This reliance assumes a static wager by the player as well as all of the variables remains constant. There is a branch of probability mathematics that challenges this idea and it is the chaos theory of mathematics.
The simplest way to illustrate chaos theory is through a fractal geometry image. In its simplest form chaos theory asserts that a seemingly random line along a geometric plane, when viewed in a larger context (or zoomed out view on a geometric plane), displays a discernable pattern. Another key concept is that the small changes within a closed system leads to drastic changes downstream from that event. The classic example is that a butterfly flaps its wings in the jungle and causes a car crash in new york.
The mathematical derivation of these assertions is pretty complex and well beyond the understanding of most recreational and pro gamblers. This article will strictly focus on how gamblers can benefit from even a basic understanding of how it can be applied.

BLACKJACK CHAOS THEORY
Every game has a set of rules that dictate the overall advantage the casino enjoys. Blackjack rules can vary by whether you can double after the split of if the dealer stands or hits on a soft 17. To determine what the house edge is billions of computer simulations are utilized to find a convergence of a loss per dollar using an optimal strategy and a flat bet for each event. Blackjack is used the first example because its system is a dependent one not based on the law of independent trials. For instance, when an ace is played then there is one less ace in the deck available for a blackjack. But it is a good lead into evaluating games that are more accepted as adhering to the law of independent trials.
Blackjack is a dynamic game and what cards are played in prior hands directly affect what hands will be played in the future. Each card played either adds to or subtracts from the houses initial advantage. Ans when enough of the right cards are dealt the advantage swings to the player’s advantage. The players then up their bets and can gain the advantage over the house. Other games offered by casinos are also susceptible to this type of attack. The dynamics are much more subtle but they are there. And as stated before a defining characteristic of chaos theory is that small changes cause drastic down-stream results. Roulette is the most common application of this.

American roulette wheels have 38 spaces; 1-36 and a 0 and 00 slot. In a perfect statistical world, the ball would land in each basket an average of 1 time in 38. This would indicate that the conditions that the ball was initially spun in remain the same for every spin. This is not the case. The wheel inevitably experiences wear and tear. The humidity in the casino changes over time. The temperature of the wheel will change depending how many people are in the casino. All of these differences may seem unimportant to the recreational player, but as chaos theory teaches us small changes leads to drastic shifts in the end results.
Now that it is clear what the small changes are that can lead to a shift the question becomes what is the end result and how can we determine the end result and the most important question how can a knowledgeable player take advantage of the information for financial gain.
The first question is what is the end result? The clear result is that some numbers will come up more frequently than others, creating a number bias. So how is it that a player can determine what the bias is. Unfortunately, there is no short way to determine this. The only way is to collect data; more precisely a whole lot of data. For roulette, no less than 50,000 spins on a given roulette wheel should be evaluated. A roulette wheel spins approximately 20 times per hour. It is very time-consuming to get the initial data set. This begs the question what is the best approach for data collection?

Because not every wheel will have a biased it is best to record data for several wheels at one time. The data should be put into a spreadsheet and evaluated to see what the frequency of each number is. A one time in 36 frequency yields a positive game and any frequency greater than 1 time 36 is a positive expectation game. Any time you can find at least 2 bias wheels in 10 is a great find. There are both good and bad aspects of this methodology.
First, the bad, the obvious downs side is it takes a very long time to collect the data, several months in fact. Once the data is collected it takes several more months to utilize the system and realize the positive expectation. It is perfectly reasonable that a negative downswing can be experienced during the first few weeks, thus leading to an emotional turmoil. Another thing to consider is that a casino can change out the wheel at any time rendering all your data collection useless.
Now the good, the financial value of this approach is fantastic if you find a wheel with frequencies occurring any more frequent than 1 time in 25. Betting numbers on the inside, as well as ranges and red and black all, can be utilized in this approach. Once you know what the long-term outcome will be you can take full advantage of this information for an exceptional profit.
CHAOS THEORY IN ONLINE CASINOS
In the online realm, data can also be collected to determine if there is any bias in the random number generators. There is almost always a slight bias in all RNG’s, however, the bias can only be determined after 100 of thousands of events. The chaos theory application can be applied to online baccarat through this approach. However, it cannot be used on traditional table games because the casinos only use the baccarat cards one time before they are no longer used.
The chaos theory is all about taking advantage of small unnoticeable changes in a system. It is a tedious process but when applied correctly it is a very profitable approach to casino gaming.
Poker game theory
Game theory, specifically for skill based casino games, is the advanced study of game playing strategies. The central idea is finding the best possible methods with which to defeat opponents. Not just any opponents, however, but opponents who themselves may have studied game theories or strategies of their own. The idea is to outsmart a person who is as skilled as the player, him-, or her-, self.
The primary focus of much game theory is on poker, be it online or real world. The reason for this is that poker is by far the most skill based casino game, being that its focus is primarily on playing against other, real world opponents. Multiplayer online poker is a prime example, given that your opponents are made of other players who may be familiar with poker strategies. Let’s have a look at 5 essential points of interest in game theory versus real world poker opponents.
1.There is no perfect strategy
One of the most important things to keep in mind is that there is no such thing as a perfect strategy. All casino games, including poker, are games that are based on chance, and this unknown factor means that all strategies are variable in nature. All strategies aim to deal with the unknown factor as best as possible, but there is no one answer that will fit all situations in a perfect manner.
So what does this mean? It means that regardless of how knowledgeable any player is with game theories or strategies, they will always find themselves in situations where there is no definite move that will guarantee positive results. In other words; it is impossible to become a perfect player of a game such as poker. One can only hope to be as good as is possible, with the knowledge available, and as much practice as can be managed.
2.The twin approach
Game theory, as already mentioned, focuses on playing a variable game against an opponent who is assumed to be equally as skilled. This is sometimes referred to as the twin approach, given that theorist are fond of saying that a genius versus his exact twin would be the most interesting poker game in history. But how does one defeat an equally skilled opponent at a game such as poker?
The game theory in this scenario is much like a game of chess, but with a board that rearranged itself at the end of every turn. That is to say; it requires a great deal of prediction, adaptability, and a thorough understanding of statistical chance. The only real way to defeat one’s twin would be to act in thoroughly unpredictable and unexpected ways, while keeping the goal of victory clearly in mind.
3.Zero-sum games
Poker is referred to as a zero-sum game. This means that at the end of each round of poker, all bets that have been placed will end up in one lucky person's possession. The amount of the winnings will have been lost by the opposing players. The object of the game is to gather enough of the flexible resource that the other players are sitting with zero.
Game theory deals with the concept, in part, that the flexible resource, gambling chips, may drastically sway the way player approach the game. A player with more chips will behave differently to one who has very few remaining. This is an aspect often overlooked by many strategies, and something that may throw off even professional, highly experienced, players.
4.Perfect information game
Poker is a referred to as a perfect information game. This means that every player in the game has the exact same information as every other player, beyond the values of their own cards. There is no move a player can make that is unseen, or unknown by any other player.
This means that the results of poker are greatly determined by how each player interprets each move made. In other words, there are few games that are as fair, and based on individual skill, as poker.
5.Optimal strategies
It is said that if two players go head to head in a game of poker, and one player uses an optimal strategy, he or she may even tell the opposing player what they are doing in each step, and will still end up as the victor. This simple idea explains the very core of game theory. That is to say; an optimal strategy is effective, regardless of how much information the opposing player has.
As has already been said, such a strategy is virtually impossible, but is the ultimate goal, in principal, of game theorists. The best a player can do until a mythical optimal strategy is achieved is use a number of flexible strategies in the best manner possible. And, most importantly, not keep using the same strategy, which will avoid it becoming predictable.
The world of information and game theory
Information theory created the framework around which both the modern digital world, and card counting in casinos, are based.
Turning decision making into a science rather than an art form was process that developed from around the 1940s onward in response to a problem all telephone companies had with long distance communications. Build a long telephone wire and the quality degrades over distance, stick an amp half way and the signal will still be strong, but you’ll be amplifying the distortion too.
Shannon and his cohorts developed a system whereby all information was turned into a positive or negative state that could be used to transmit the symbology of all communication. It didn’t matter if it were words, numbers, music, imagery, whatever, it could all be encoded down into a series of bits. These bits (single pieces of yes/no information) became the common denominator of information.
Bits revolutionized communications… then the world. The microprocessor you’re using to read this, the internet you got it from, the drive you might store it on and indeed any compression you care to use to make it smaller, they all still work on binary information, they still work on bits. At the time this was a paradigm shift in thinking that some have compared to the work of einstein.
Rational betting through game theory
• modern world based on information theory
• bits connecting the pieces
Shannon’s pursuit of digital logic in the late 1940s is directly responsible for most of the modern technology we now take entirely for granted. It is even responsible for the manner in which a great many people spend their time winning at blackjack. Counting cards is merely information theory used in a very specific way.
Game theory
John larry kelly jnr worked for claude shannon at the bell telephone lab and felt “information theory can be thought of as a way of quantifying information so as to make the best decision in the face of imperfect information” and one of the situations in which there was imperfect information was at the blackjack table.
The history of blackjack is full of people with “a system” but perhaps for the first time a mathematical basis was applied not to winning a single bet or hand, but to win over the long term growing the bankroll of the player. This fractional betting of payroll based on the maximization of opportunity as against risk is still the basis of card counting strategies.
The mathematical formulas of information theory are now being applied to the relatively simple construct of a card game, and the pursuit of the best possible response to each circumstance. This is regardless of decisions made by the opposition, the dealer, because there’s no control over that side of the situation.
The kelly criterion is an established and proven theory of not just gambling per se but of any speculative action. The application of these theories and formulas has been as successful on wall street as they have in las vegas or, indeed, the laboratory. Game theory is merely the part of information theory that explores how we use information for our own advantage.
Prisoner’s dilemma
The idea that there is a logical basis for decision making despite imperfect information was best summed up in the prisoners dilemma, an example of game theory. Two prisoners held apart and incommunicado must choose to either betray their fellow prisoner or stay silent. Each possible permutation of their actions has a different outcome.
If they both betray each other they’ll get both get 2 years in the chokey. If either one betrays the other they’ll be set free but the other will serve 5 years inside. And if they both stay schtum they’ll only get 1 year each in prison on a lesser charge. So now what is the best course of action for each one?
Well if both are purely rational the logical thing to do is for both to betray each other, because that is the promotion of self-interest. The betrayal of the other is the best course of action regardless of the other prisoner’s action. Either you get 2 years each for betraying each other, or if the other prisoner didn’t you’re going to go free. This is better than the possible 5 year stretch you’ll be doing if you don’t.
This example of game theory is quite esoteric as it denies the possibility of cooperation (something humans tend towards most of the time) and also demonstrates that you should never commit a crime with a scientist or mathematician because they’ll grass on you. In the real world game theory of this nature is applied to business in wall street just as much as it is to blackjack in asia. Rational choice made from known data.
Chaos theory: can it become a strategy at blackjack & roulette?


The law of independent trials is fundamental principle that allows the casino gaming industry to accumulate mass amounts of wealth from unsuspecting customers. Every game in the casino has the perception of being completely random in nature. This means there is no predictability of the outcome of an upcoming event. What the casinos rely on is the overall advantage that their games have that favor the casino. This reliance assumes a static wager by the player as well as all of the variables remains constant. There is a branch of probability mathematics that challenges this idea and it is the chaos theory of mathematics.
The simplest way to illustrate chaos theory is through a fractal geometry image. In its simplest form chaos theory asserts that a seemingly random line along a geometric plane, when viewed in a larger context (or zoomed out view on a geometric plane), displays a discernable pattern. Another key concept is that the small changes within a closed system leads to drastic changes downstream from that event. The classic example is that a butterfly flaps its wings in the jungle and causes a car crash in new york.
The mathematical derivation of these assertions is pretty complex and well beyond the understanding of most recreational and pro gamblers. This article will strictly focus on how gamblers can benefit from even a basic understanding of how it can be applied.

BLACKJACK CHAOS THEORY
Every game has a set of rules that dictate the overall advantage the casino enjoys. Blackjack rules can vary by whether you can double after the split of if the dealer stands or hits on a soft 17. To determine what the house edge is billions of computer simulations are utilized to find a convergence of a loss per dollar using an optimal strategy and a flat bet for each event. Blackjack is used the first example because its system is a dependent one not based on the law of independent trials. For instance, when an ace is played then there is one less ace in the deck available for a blackjack. But it is a good lead into evaluating games that are more accepted as adhering to the law of independent trials.
Blackjack is a dynamic game and what cards are played in prior hands directly affect what hands will be played in the future. Each card played either adds to or subtracts from the houses initial advantage. Ans when enough of the right cards are dealt the advantage swings to the player’s advantage. The players then up their bets and can gain the advantage over the house. Other games offered by casinos are also susceptible to this type of attack. The dynamics are much more subtle but they are there. And as stated before a defining characteristic of chaos theory is that small changes cause drastic down-stream results. Roulette is the most common application of this.

American roulette wheels have 38 spaces; 1-36 and a 0 and 00 slot. In a perfect statistical world, the ball would land in each basket an average of 1 time in 38. This would indicate that the conditions that the ball was initially spun in remain the same for every spin. This is not the case. The wheel inevitably experiences wear and tear. The humidity in the casino changes over time. The temperature of the wheel will change depending how many people are in the casino. All of these differences may seem unimportant to the recreational player, but as chaos theory teaches us small changes leads to drastic shifts in the end results.
Now that it is clear what the small changes are that can lead to a shift the question becomes what is the end result and how can we determine the end result and the most important question how can a knowledgeable player take advantage of the information for financial gain.
The first question is what is the end result? The clear result is that some numbers will come up more frequently than others, creating a number bias. So how is it that a player can determine what the bias is. Unfortunately, there is no short way to determine this. The only way is to collect data; more precisely a whole lot of data. For roulette, no less than 50,000 spins on a given roulette wheel should be evaluated. A roulette wheel spins approximately 20 times per hour. It is very time-consuming to get the initial data set. This begs the question what is the best approach for data collection?

Because not every wheel will have a biased it is best to record data for several wheels at one time. The data should be put into a spreadsheet and evaluated to see what the frequency of each number is. A one time in 36 frequency yields a positive game and any frequency greater than 1 time 36 is a positive expectation game. Any time you can find at least 2 bias wheels in 10 is a great find. There are both good and bad aspects of this methodology.
First, the bad, the obvious downs side is it takes a very long time to collect the data, several months in fact. Once the data is collected it takes several more months to utilize the system and realize the positive expectation. It is perfectly reasonable that a negative downswing can be experienced during the first few weeks, thus leading to an emotional turmoil. Another thing to consider is that a casino can change out the wheel at any time rendering all your data collection useless.
Now the good, the financial value of this approach is fantastic if you find a wheel with frequencies occurring any more frequent than 1 time in 25. Betting numbers on the inside, as well as ranges and red and black all, can be utilized in this approach. Once you know what the long-term outcome will be you can take full advantage of this information for an exceptional profit.
CHAOS THEORY IN ONLINE CASINOS
In the online realm, data can also be collected to determine if there is any bias in the random number generators. There is almost always a slight bias in all RNG’s, however, the bias can only be determined after 100 of thousands of events. The chaos theory application can be applied to online baccarat through this approach. However, it cannot be used on traditional table games because the casinos only use the baccarat cards one time before they are no longer used.
The chaos theory is all about taking advantage of small unnoticeable changes in a system. It is a tedious process but when applied correctly it is a very profitable approach to casino gaming.
Game theory in 10 easy steps
1. AS YOU MIGHT HAVE GUESSED, “GAME THEORY” IS THE MATHEMATICAL STUDY OF GAMES
But not just any type of game. Game theory concerns only games of strategy between two or more competing players. Usually there’s a clear set of rules that define the possible moves that each player can make at each juncture in the game and a resulting score for each player at the end of each play of the game.
2. DON’T CONFUSE “GAME THEORY” WITH “GAMBLING THEORY”
Although the two do overlap. Gambling theory applies to games with uncertain outcome, with one or many players, with or without any skill involved. You could say that game theory is about games of skill, and gambling theory about games of chance (although a single-player gambling game that involves some skill would not be a subject of game theory). Video poker, which involves just a single player attempting to make winning combinations, has an element of skill but it still wouldn’t be a subject for game theory. But live poker, played amongst two or more people, is a fitting subject for game theory. In fact, poker games, especially simplified versions, have been used as paradigms in game theory since john von neumann founded modern game theory back in the 1920s.
Similarly, game theory doesn’t apply to the game of blackjack, even when a skillful card counter gains an edge over the casino. But, if you consider the cat-and-mouse game that card counters have to excel at to be allowed to keep playing and beating the casino, game theory has a lot to say about that.
3. GAME THEORY SPLITS UP GAMES INTO TWO TYPES ALONG SEVERAL DIFFERENT CRITERIA
There are cooperative versus non-cooperative games, zero-sum (or more accurately, constant-sum) versus non-zero-sum games, simultaneous versus sequential games, games of perfect information versus games of imperfect information, and two-player games versus games with three or more player. There are also symmetric and asymmetric games, infinite games, combinatorial games, repeated games, discrete or continuous games, population games, stochastic games, meta-games, and so on.
It’s good to know that these terms exist, but I won’t be able to cover all of them here.
4. THE ECONOMIC PREDICTING BEHAVIOR MODEL
Game theory was originally developed as a mathematical model for studying and predicting economic behavior. But the wide variety of games that game theory contemplates makes it valuable as a model or tool for many fields, including evolutionary biology, philosophy, political science, computer science, warfare, and, of course, multi-person gambling games like poker. Remember the classic 1983 movie war-games? (if you haven’t seen it, that’s your first homework assignment.) that’s game theory applied to thermonuclear war.
“perfect information” in game theory doesn’t mean that you know the future. It only means that each player has perfect information about the past
5. I WANT TO PLAY A (ZERO SUM) GAME
A game is zero-sum if the total score at the end of each play is zero. In other words, however much one person wins will be lost by the other player(s) in the game. Gambling games like poker are usually zero-sum games (not including the house rake, but also not including the entertainment value, which is why we play in the first place). There are some games where players can combined do better or worse depending on their strategies. Games like this are used sometimes as models for how cooperation can evolve between otherwise competing players.
6. CAN YOU PREDICT THE FUTURE? THAT’S PERFECT
“perfect information” in game theory doesn’t mean that you know the future. It only means that each player has perfect information about the past (and present, if there are simultaneous moves by different players). There’s no hidden information that one player knows that another doesn’t, like a player’s hidden cards in a poker game. And there are no hidden moves, like the starting position in a game of battleship.
Games like chess, checkers, and go are games of perfect information, and there’s a separate branch of mathematics called combinatorial game theory that focuses on them. “regular” game theory focuses more on games with imperfect information. There still can be randomness and uncertainty in a game of perfect information. Backgammon is a good example, and monopoly too. (speaking of monopoly and war games, in world war II, the allies actually smuggled maps, compasses, and other tools to pows, inside monopoly boards!)
7. IT’S ALL ABOUT INELEGANCE
The main focus of game theory is on fully rational, intelligent players.
Game theory is interested in answering the question, “what’s the best way to play against opponents that think like I do?” the focus isn’t on how to play against a particular opponent with a certain strategy (although that can be a step in an algorithm for finding an optimal strategy). It’s like a genius playing against a perfect twin.
“what’s the best way to play against opponents that think like I do?” the focus isn’t on how to play against a particular opponent with a certain strategy.
8. PURE STRATEGY VS MIXED STRATEGY
In every two-player zero-sum game, like heads-up poker, there is a strategy (or a set of strategies) that cannot be beaten, even by the best opponent. That’s usually called the optimal strategy. If you follow the optimal strategy, you can even tell your opponent how you play (but don’t give away tells!) and you can’t be beaten in the long run. Optimal strategies often involve picking randomly (but with precise probabilities) between two or more different playing choices for some or all moves of the game, because an opponent can sometimes take advantage of a strategy that is too predictable. (that’s called a “mixed strategy” in game theory, as opposed to a “pure strategy” that always makes the same choice of move in identical situations.)
for example, in the game rock-paper-scissors (also called roshambo), the optimal strategy is to make each play one-third of the time. If you played rock even slightly more often than that, your opponent could have an advantage by playing paper more often than one third and/or playing scissors less often. Note that the optimal strategy in roshambo won’t give you an advantage, but it will prevent your opponent from gaining an advantage. Fortunately, that’s not true of poker. The optimal strategy, if you can figure it out and follow it perfectly, will give you an advantage against almost anyone else.
You may have heard that poker researchers “solved” a real-life poker game last year (heads-up limit texas hold’em). They didn’t literally solve the game, but they did get close enough, using thousands of days of computer time, that they felt they could say that they “essentially” solved it. You could play perfectly against it for your whole life and still have a good chance of losing.
Quote: if you follow the optimal strategy, you can even tell your opponent how you play (but don’t give away tells!) and you can’t be beaten in the long run
9. EQUILIBRIUM STRATEGIES
Add just one more player, so there are 3 players in a game (or the game isn’t zero-sum) and there isn’t guaranteed to be a “best” or “optimal” way to play. However, there can be “equilibrium strategies”, called nash equilibria, named after john nash, the famous mathematician who won the nobel prize in economics in 1994 for his groundbreaking work. Nash was the subject of the multiple-academy award-winning movie A beautiful mind, based on the book of the same name. Sadly, last year he and his wife were killed in a car accident in new jersey, being driven home from the airport after another prize-winning trip to norway.
10. GET OUT THERE AND PLAY
In a nash equilibrium, no player can do better by unilaterally changing his or her strategy. But, two or more players could change their strategy together and see an improvement. Applying that to a poker game: when there are more than 2 players in the game, there is no strategy that will always win (even when your opponents aren’t purposefully colluding against you). That means you must adapt your strategy to your opponents, and how they are playing at that time. To complicate matters, they are going to be constantly trying to adapt to you and each other too. And, sometimes, even by chance, they may be playing in a way that you can’t beat in the long run. So, find a poker game that you’re comfortable in and can win at, or seek a heads-up (two player) game.
Finally, that brings us to your final assignment: get out there and play. (tell ‘em you’re just doing your homework!)
Quantitative trading
Game theory
We’ve got game.
Game theory rings true in everything we do. We’re big on competition, strategy, and managing risk – just like great gamers are. Find out how we use our favorite games to hone our skills and apply them to complex financial markets.





The game of poker is valuable in more ways than you may think. We use poker to teach new traders about decision making under uncertainty. Our traders go through similar thought processes while evaluating the expected value of a given trade and deciding how to price risk.

We’ve found that the best strategy does not necessarily depend on what has already happened in the game, but how past actions of your opponents can provide insight into the way they think.
We challenged jennifer shahade, two-time american women’s chess champion, to a simul chess game. See the match results



Magic requires the same set of skills our traders use: decision making with imperfect information, observation of your opponents, and strategizing to maximize your potential to win. It’s kind of a big deal here, and so are some of our players.



We like board games that require strategic thinking, pattern identification, and quick response time. We like the challenge of backgammon, the settlers of catan, SET, the resistance, hanabi, power grid, and dominion – just to name a few.

Strategy is a huge part of certain video games. We like action games that require reactions to small changes in a fast paced environment.

There are many lessons that carry over from team sports to SIG. We work collaboratively, each playing his or her part in information gathering, assessing alternatives, execution, and risk management.
See how we team up outside of work for our under 30 vs. Over 30 soccer match
Economic and game theory
what is game theory?
Top |general | research | classes | links | david
What economists call game theory psychologists call the theory of social situations, which is an accurate description of what game theory is about. Although game theory is relevant to parlor games such as poker or bridge, most research in game theory focuses on how groups of people interact. There are two main branches of game theory: cooperative and noncooperative game theory. Noncooperative game theory deals largely with how intelligent individuals interact with one another in an effort to achieve their own goals. That is the branch of game theory I will discuss here.
In addition to game theory, economic theory has three other main branches: decision theory, general equilibrium theory and mechanism design theory. All are closely connected to game theory.
Decision theory can be viewed as a theory of one person games, or a game of a single player against nature. The focus is on preferences and the formation of beliefs. The most widely used form of decision theory argues that preferences among risky alternatives can be described by the maximization of the expected value of a numerical utility function, where utility may depend on a number of things, but in situations of interest to economists often depends on money income. Probability theory is heavily used in order to represent the uncertainty of outcomes, and bayes law is frequently used to model the way in which new information is used to revise beliefs. Decision theory is often used in the form of decision analysis, which shows how best to acquire information before making a decision.
General equilibrium theory can be viewed as a specialized branch of game theory that deals with trade and production, and typically with a relatively large number of individual consumers and producers. It is widely used in the macroeconomic analysis of broad based economic policies such as monetary or tax policy, in finance to analyze stock markets, to study interest and exchange rates and other prices. In recent years, political economy has emerged as a combination of general equilibrium theory and game theory in which the private sector of the economy is modeled by general equilibrium theory, while voting behavior and the incentive of governments is analyzed using game theory. Issues studied include tax policy, trade policy, and the role of international trade agreements such as the european union.
Mechanism design theory differs from game theory in that game theory takes the rules of the game as given, while mechanism design theory asks about the consequences of different types of rules. Naturally this relies heavily on game theory. Questions addressed by mechanism design theory include the design of compensation and wage agreements that effectively spread risk while maintaining incentives, and the design of auctions to maximize revenue, or achieve other goals.
An instructive example
One way to describe a game is by listing the players (or individuals) participating in the game, and for each player, listing the alternative choices (called actions or strategies) available to that player. In the case of a two-player game, the actions of the first player form the rows, and the actions of the second player the columns, of a matrix. The entries in the matrix are two numbers representing the utility or payoff to the first and second player respectively. A very famous game is the prisoner's dilemma game. In this game the two players are partners in a crime who have been captured by the police. Each suspect is placed in a separate cell, and offered the opportunity to confess to the crime. The game can be represented by the following matrix of payoffs
| Not confess | confess | |
| not confess | 5,5 | -4,10 |
| confess | 10,-4 | 1,1 |
Note that higher numbers are better (more utility). If neither suspect confesses, they go free, and split the proceeds of their crime which we represent by 5 units of utility for each suspect. However, if one prisoner confesses and the other does not, the prisoner who confesses testifies against the other in exchange for going free and gets the entire 10 units of utility, while the prisoner who did not confess goes to prison and which results in the low utility of -4. If both prisoners confess, then both are given a reduced term, but both are convicted, which we represent by giving each 1 unit of utility: better than having the other prisoner confess, but not so good as going free.
This game has fascinated game theorists for a variety of reasons. First, it is a simple representation of a variety of important situations. For example, instead of confess/not confess we could label the strategies "contribute to the common good" or "behave selfishly." this captures a variety of situations economists describe as public goods problems. An example is the construction of a bridge. It is best for everyone if the bridge is built, but best for each individual if someone else builds the bridge. This is sometimes refered to in economics as an externality. Similarly this game could describe the alternative of two firms competing in the same market, and instead of confess/not confess we could label the strategies "set a high price" and "set a low price." naturally it is best for both firms if they both set high prices, but best for each individual firm to set a low price while the opposition sets a high price.
A second feature of this game, is that it is self-evident how an intelligent individual should behave. No matter what a suspect believes his partner is going to do, it is always best to confess. If the partner in the other cell is not confessing, it is possible to get 10 instead of 5. If the partner in the other cell is confessing, it is possible to get 1 instead of -4. Yet the pursuit of individually sensible behavior results in each player getting only 1 unit of utility, much less than the 5 units each that they would get if neither confessed. This conflict between the pursuit of individual goals and the common good is at the heart of many game theoretic problems.
A third feature of this game is that it changes in a very significant way if the game is repeated, or if the players will interact with each other again in the future. Suppose for example that after this game is over, and the suspects either are freed or are released from jail they will commit another crime and the game will be played again. In this case in the first period the suspects may reason that they should not confess because if they do not their partner will not confess in the second game. Strictly speaking, this conclusion is not valid, since in the second game both suspects will confess no matter what happened in the first game. However, repetition opens up the possibility of being rewarded or punished in the future for current behavior, and game theorists have provided a number of theories to explain the obvious intuition that if the game is repeated often enough, the suspects ought to cooperate.
If we were all better people the world would be A better place
Some of the power and meaning of game theory can be illustrated by assessing the statement "if we were all better people the world would be a better place." this may seem to you to be self-evidentally true. Or you may recognize that as a matter of logic this involves the fallacy of composition: just because a statement applies to each individual person it need not apply to the group. Game theory can give precise meaning to the statement of both what it means to be better people and what it means for the world to be a better place, and so makes it possible to prove or disprove the statement. In fact the statement is false, and this can be shown by a variation of the prisoner's dilemma.
Let us start with a variation on the prisoner's dilemma game we may call the pride game.
| | Proud | not confess | confess |
| proud | 4.0, 4.0 | 5.4, 3.6 | 1.2, 0.0 |
| not confess | 3.6, 5.4 | 5.0, 5.0 | -4.0, 10.0 |
| confess | 0.0, 1.2 | 10.0, -4.0 | 1.0, 1.0 |
The pride game is like the prisoner's dilemma game with the addition of the new strategy of being proud. A proud individual is one who will not confess except in retaliation against a rat-like opponent who confesses. In other words, if I stand proud and you confess, I get 1.2, because we have both confessed and I can stand proud before your humiliation, but you get 0, because you stand humiliated before my pride. On the other hand, if we are both proud, then neither of us will confess, however, our pride comes at a cost, as we both try to humiliate the other, so we each get 4, rather than the higher value of 5 we would get if we simply chose not to confess. It would be worse, of course, for me to lose face before your pride by choosing not to confess. In this case, I would get 3.6 instead of 4, and you, proud in the face of my humiliation would get 5.4.
The pride game is very different than the prisoner's dilemma game. Suppose that we are both proud. In the face of your pride, if I simply chose not to confess I would lose face, and my utility would decline from 4 to 3.6. To confess would be even worse as you would retaliate by confessing, and I would be humiliated as well, winding up with 0. In other words, if we are both proud, and we each believe the other is proud, then we are each making the correct choice. Morever, as we are both correct, anything either of us learns will simply confirm our already correct beliefs. This type of situation - where players play the best they can given their beliefs, and they have learned all there is to learn about their opponents' play is called by game theorists a nash equilibrium .
Notice that the original equilibrium of the prisoner's dilemma confess-confess is not an equilibrium of the pride game: if I think you are going to confess, I would prefer to stand proud and humiliate you rather than simply confessing myself.
Now suppose that we become "better people." to give this precise meaning take this to mean that we care more about each other, that is, we are more altruistic, more generous. Specifically, let us imagine that because I am more generous and care more about you, I place a value both on the utility I receive in the "selfish" game described above and on the utility received by you. Not being completely altruistic, I place twice as much weight on my own utility as I do on yours. So, for example, if in the original game I get 3 units of utility, and you get 6 units of utility, then in the new game in which I am an altruist, I get a weighted average of my utility and your utility. I get 2/3 of the 3 units of utility that belonged to me in the original "selfish" game, and 1/3 of the 6 units of utility that belonged to you in the "selfish" game. Overall I get 4 units of utility instead of 3. Because I have become a better more generous person, I am happy that you are getting 6 units of utility, and so this raises my own utility from the selfish level of 3 to the higher level of 4. The new game with altruistic players is described by taking a weighted average of each player's utility with that of his opponent, placing 2/3 weight on his own utility and 1/3 weight on his opponent's. This gives the payoff matrix of the altruistic pride game
| | Proud | not confess | confess |
| proud | 4.00, 4.00 | 4.8, 4.20* | 0.80, 0.40 |
| not confess | 4.20*, 4.80 | 5.00, 5.00 | 0.67, 5.33* |
| confess | 0.40, 0.80 | 5.33*, 0.67 | 1.00*, 1.00* |
What happens? If you are proud, I should choose not to confess: if I were to be proud I get a utility of 4, while if I choose not to confess I get 4.2, and of course if I do confess I get only 0.4. Looking at the original game, it would be better for society at large if when you are proud I were to choose not to confess. This avoids the confrontation of two proud people, although of course, at my expense. However, as an altruist, I recognize that the cost to me is small (I lose only 0.4 units of utility) while the benefit to you is great (you gain 1.4 units of utility), and so I prefer to "not confess." this is shown in the payoff matrix by placing an asterisk next to the payoff 4.2 in the proud column.
What should I do if you choose not to confess? If I am proud, I get 4.8, if I choose not to confess I get 5, but if I confess, I get 5.33. So I should confess. Again, this is marked with an asterisk. Finally, if you confess, then I no longer wish to stand proud, recognizing that gaining 0.2 by humiliating you comes at a cost of 1 to you. If I choose not to confess I get only 0.67. So it is best for me to confess as well.
What do we conclude? It is no longer an equilibrium for us both to be proud. Each of us in the face of the other's pride would wish to switch to not confessing. Of course it is also not an equilibrium for us both to choose not to confess: each of us would wish to switch to confessing. The only equilibrium is the box marked with two asterisks where we are both playing the best we can given the other player's play: it is where we both choose to confess. So far from making us better off, when we both become more altruist and more caring about one another, instead of both getting a relatively high utility of 4, the equilibrium is disrupted, and we wind up in a situation in which we both get a utility of only 1. Notice how we can give a precise meaning to the "world being a better place." if we both receive a utility of 1 rather than both receiving a utility of 4, the world is clearly a worse place.
The key to game theory and to understanding why better people may make the world a worse place is to understand the delicate balance of equilibrium. It is true that if we simply become more caring and nothing else happens the world will at least be no worse. However: if we become more caring we will wish to change how we behave. As this example shows, when we both try to do this at the same time, the end result may make us all worse off.
To put this in the context of day-to-day life: if we were all more altruistic we would choose to forgive and forget more criminal behavior. The behavior of criminals has a complication. More altruistic criminals would choose to commit fewer crimes. However, as crime is not punished so severely, they would be inclined to commit more crimes. If in the balance more crimes are committed, the world could certainly be a worse place. The example shows how this might work.
For those of you who are interested in or already know more advanced game theory, the pride game has only the one nash equilibrium shown - it is solvable by iterated strict dominance. The atruistic pride game, however, has several mixed strategy equilibria. You can compute them using the fine open source software program gambit written by richard mckelvey, andrew mclennan and theodore turocy. One equilibrium involves randomizing between proud and confess, so is worse than the proud-proud equilibrium of the pride game. The other is strictly mixed in that it randomizes between all three strategies. The payoffs to that equilibrium gives each player 2.31 - so while it is better than both players confessing for certain, it is still less good than the unique equilibrium of the pride game.
I'd like to thank jie zheng for his help. The "we are all better people" example is inspired by and based on an academic paper by sung-ha hwang and samuel bowles called "is altruism bad for cooperation?" if you know some basic calculus the paper is very readable, and I will provide a link as soon as they post it online. They provide a much more persuasive and robust example of how altruism hurts cooperation, tightly linked to experimental evidence. If you wish to learn more about game theory, there a variety of good books on the topic.
So, let's see, what we have: explore the field of game theory and see how it can equip you for daily decision-making. At casino game theory
No comments:
Post a Comment